Riemann for Anti-Dummies Part 62

On the Continuum of the Discontinuum by Bruce Director

In the Laws, Plato's Athenian stranger laments that the Greek people's pervasive ignorance of the incommensurability of a line with a surface and a surface with a solid, had rendered them more like "guzzling swine" than true human beings. While the same lament can be sounded with respect to today's society, modern citizens must also include an understanding of those higher transcendentals which are the subject of Riemann's "Theory of Abelian Functions", if they are to avoid the lamentable fate of Plato's fellow Greeks. To rectify this condition, Plato recommended that these subjects should be learned in childhood, through play. Yet those who, today, have already graduated from that stage in life should, nevertheless, not despair. For them such knowledge can still be assimilated through that form of fun that has come to be known as pedagogical exercises, provided that one approaches this play with the zeal and eagerness of youth. What Plato considered most important to glean from this play is not the facts (such as, that an area can not be measured by a line, or that a volume can not be measured by an area), but the cognitive experience of discovering the underlying principle implied by the incommensurability of these three different types of action. Through this experience the mind recognizes its power to recognize what Plato called "powers", so that when confronted with "facts", it recognizes, as Riemann did, that all facts are hypotheses, and it is attracted not to the factual statements by themselves, but to the underlying principles on which the hypotheses, implied by the existence of such facts, are based. These principles, thus recognized as thought-objects, become a proper, and necessary, subject for further investigation. From investigations typified by the discovery of the incommensurability of the line, square and cube, we learn of the relationship between objects of sense and the underlying principles that govern their characteristics. For the relationships among principles and those relationships to the mind, we must turn to the subject of Riemann's "Theory of Abelian Functions". From that standpoint, let us continue our pedagogical investigation of Riemann's work.

Discreteness and Continuity

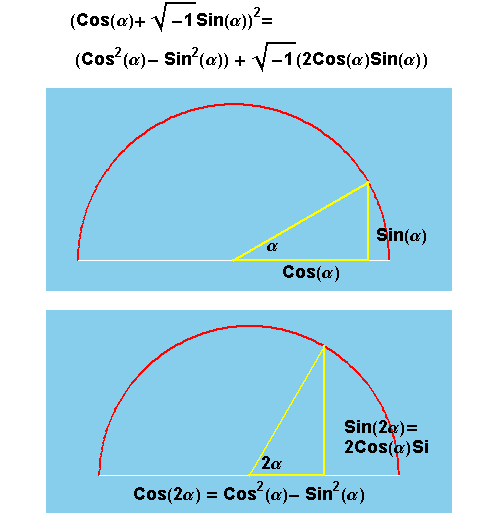
While the principles for the first type of investigation were well established by the Pythagorean science of Sphaerics, to which Plato's comments refer, their most general, modern elaboration was laid down by Riemann in his 1854 habilitation dissertation. Generalizing the method that had been developed by the successive accomplishments of Cusa, Kepler, Leibniz and Gauss, Riemann set forth the fundamental basis by which scientific investigations into the effect of physical principles on the objects of sense must proceed--without the anti-scientific presumption of the {a priori} axioms, postulates and definitions of Euclidean geometry. Riemann rejected Kant's insistence that the characteristics of Euclidean geometry were "hard- wired" into the human mind. Instead of this Aristotelean dogma, Riemann recognized that the mind understands physical processes by forming concepts of a multiply-extended manifold whose characteristics are determined by physical principles, or "modes of determination" (Bestimungsweissen) that govern that action. These modes of determination can never be given {a priori}, but must be discovered by the unique experiments that test the underlying hypotheses on which the concept of the manifold is based. Thus, the nature of the physical process can only be understood in relation to the essential physical characteristics of the manifold. The number of principles acting on the manifold (extension), and the metric relations determined by those principles (curvature), are identified by Riemann as such fundamental characteristics. In the {Theory of Abelian Functions}, Riemann includes the density of singularities among the essential characteristics. Again, these characteristics, cannot be given {a priori}, but are determined by the physical characteristics of the action under investigation. To illustrate this point, look at the oft-cited example of the principle of least-action as expressed by light undergoing reflection and refraction. In the former, the principle of least- action is expressed by the invariant characteristic that the path of light from the object, to the mirror, to the eye, is always the shortest distance. Because this characteristic is reflected by the equality of the angle of incidence and the angle of reflection, this least-action effect can be completely measured simply by one mode of determination, specifically the angle of incidence. Thus, the experimental investigation of reflected light can be grasped by the mind through a concept of a simply-extended manifold. However, under refraction, this same principle of least-action is expressed not by the path of shortest distance, but by the path of shortest time, which can only be completely measured by the angle of incidence, {and} the index of refraction (the difference in the effect of the density of the two media on the velocity of light). Consequently, two modes of determination are necessary to determine position with respect to refraction, and thus, comprehending refraction, requires the formation of a concept of a doubly-extended manifold. Riemann makes a further distinction between manifolds: "According as there is, or is not, a continuous transition from one to another, they form a continuous or discrete manifold; the individual modes are called, in the first case, points, in the latter case, elements of the manifold." The objects of sense comprise, as Riemann noted, a discrete manifold, in which the "principle of metric relations is implicit in the notion of the manifold" and, "comparison as to quantity occurs by counting". The objects of such a discrete manifold are distinguished from one another by their differing physical characteristics. Thus, they are not counted with respect to {a priori} considerations, but only with respect to the characteristic modes of determination of the manifold under which they are being investigated. For example, it would not be possible to count the number of persons in a room by counting the living creatures in that room with four legs. (Unless, of course, the room included Nobel prize winning economists, or officials of the Bush Administration.) But, as Cusa and Kepler had insisted, and as Leibniz expressed it through his calculus, universal physical principles are acting continuously, albeit differentially, on the objects of sense from outside the domain of sense perception. Consequently, Riemann insisted, to comprehend the principles governing the objects of sense, we must seek the metric relationships outside the visible domain "in the colligating forces that operate upon it." Thus, for the investigation of the effect of {continuously acting physical principles} on discrete physical objects, we must form concepts of a multiply-extended continuous manifold. Riemann notes that common experience affords only a few examples of such multiply-extended continuous manifolds (the manifold of positions of objects in space and the manifold of all colors are the two examples cited by Riemann). Nevertheless, concepts of such manifolds arise when investigating physical processes that are governed by the colligating action of multiple principles. The geometrical expression for such manifolds can be formed in the complex domain, in the context of the investigation of those higher transcendentals known as "Abelian functions". A simple illustration of the principle of the latter (which is relevant for what follows), can be accomplished by rethinking one's naive concept of circular action. From the naive standpoint, the circle seems to be a simple closed curve. But what makes possible this simple circular curve? From the standpoint of Gauss and Riemann, this simple circular curve is the visible effect of the connected interaction of two continuously changing transcendental functions the sine and the cosine. Formal geometricians will find this way of thinking very uncomfortable, because from the formal standpoint, the circle seems simple and the circular functions seem complex. But, as Gauss demonstrated with his first discovery concerning the division of the circle, it is only from the standpoint of this inversion, that even the most simple propositions concerning the circle (such as the construction of the regular polygons) can be determined. Another heuristic for the same idea, is the case of the Leibniz-Bernoulli successful determination of the catenary, in contrast to Galileo's impotent pretension. Whereas Galileo fixated on attempting to describe the visible shape of the chain, Leibniz and Bernoulli investigated the continuously acting principles that determined that shape from outside the visible domain. This Leibniz -Bernoulli collaboration proved that the visible shape of the catenary was the effect of the connected interaction between the continuously changing effects of gravity and tension, the which could be measured as the arithmetic mean between two contrariwise (transcendental) exponential curves. In both these cases, the visible curves (circle and catenary) are understood as the physical effect of two, distinct but connected, modes of determination whose concept forms a doubly- extended manifold. Anther example of the method by which we investigate this relationship between continuous manifolds and their effects on the discrete objects of sense is investigated, was given by Gauss in his 1832 investigation of magnetism: "To explain magnetic phenomena, we assume two magnetic fluxes: one we call north, the other south. We presuppose, that the elements of the one flux attract those of the other, and that on the other hand, two elements of the same flux mutually repel each other, and that each of hte two effects alters in inverse relation to the square of the distance. It will be shown below that the correctness of this law was itself confirmed by our observations. "These fluxes do not occur independently, but only in association with the ponderable particles of such bodies which take on magnetism, and their effects express themselves either when they put the bodies into motion or they prevent or transform the motion, which other forces acting on these bodies, e.g. the force of gravity, would elicit. "Hence the effect of a given amount of magnetic flux on a given amount of either the same or the opposite flux at a given distance is comparable to a given motive force, i.e. with the effect of a given accelerating force on a given mass, and since the magnetic fluxes themselves can be known only through the effects, which they bring forth, the latter must directly serve to measure the former."

A Higher Continuity

But there is a still deeper expression of this relationship between discrete and continuous manifolds that will take us directly to the subject of Riemann's investigation of Abelian functions. This can be preliminarily illustrated by illuminating the above referenced investigation of Plato with light from Gauss' and Riemann's complex domain. If we think of the line, surface and volume as paradigmatic of, respectively, simply, doubly and triply extended continuous manifolds, the following paradox emerges: within each manifold there is a clear continuity of action, yet there is no continuous transition from one to the other. For example, while a point can move continuously within a line, once the line itself is moved, it forms a surface. The transition from line to surface is discontinuous. There is no in- between state where the mainfold is part line and part surface. It is either all one or all the other. Similarly, there can be continuous motion within a surface, but once the surface itself is moved, it suddenly becomes a volume. Further as Plato emphasizes, the metric relations of the line square and cube are incommensurable with each other. For example, the metric relations of a line are inadequate to measure a square. Consequently, a line adequate to measure a square, must be produced from a square, not a line. In other words, the metric relations for such a line must be sought outside the line-- in a the manifold of squares, in which lines exist only as sides of squares. Such apparent discontinuities , among continuities, point us toward a higher continuity, which is continuously acting, as a manifold of manifolds, in which these discontinuities exist simultaneously. This simultaneous continuity is not located in the manifolds themselves but in the cognitive effect of the transition from one manifold to another. Take a close look at this paradox from the standpoint of the method of Archytas for doubling the cube, in which the line, area and volume are thought of as the principles that produce, respectively, zero, one and two geometric means between two extremes.

Animated figure 1 depicts the continuous motion of a point (Q) within a simply extended manifold (line).

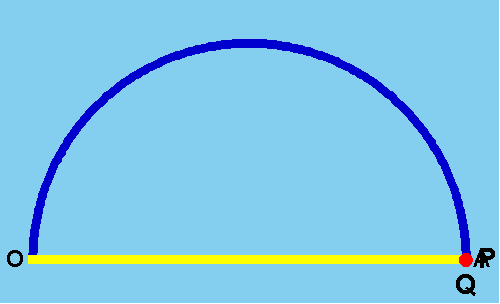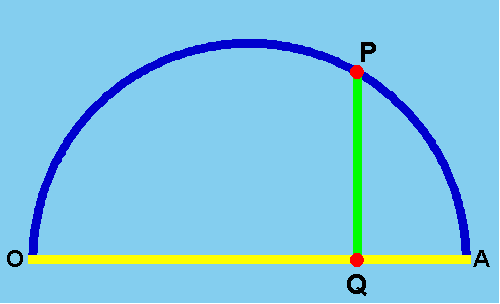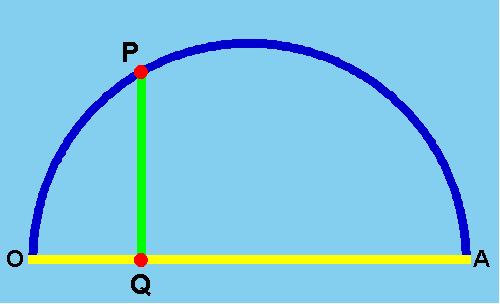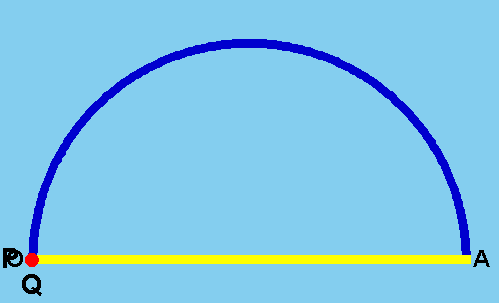
Animated figure 2 depicts that same continuous motion of (Q) but now it is occurring in a doubly-extended manifold, and is connected to the circular motion of (P).

In animated figure 3, this connected motion of figure 2 is now occurring in a triply-extended manifold, as the connected action of (P) and (Q) is now rotated within a toroidal surface.

In each case, the motion of (Q) is simply in one direction along a line, but the manifold in which that simple motion occurs increases, discontinuously, from "n" to "n+1" with the addition of each new degree of action. This effect of the addition of each new degree of action is reflected on the characteristics of the motion of (Q). In figure 1 the motion of (Q) along line AO is uniform. But when (Q) is connected to a line perpendicular to AO, whose opposite endpoint (P) is moving uniformly on a circle, (Q)'s motion along AO becomes non-uniform. Further, when line AO is rotated uniformly about (O), (P)'s motion along the circle becomes non-uniform and the non-uniform motion of (Q) along AO is changed again. The addition of each new degree of action can be measured as a change in the rate of motion of (Q) along line AO . But while the path of (Q) is always linear, its motion becomes increasingly non-linear. Further, in each case, the metric of Q's motion along line AO cannot be found with respect to Q's position within line AO, but must be sought outside line AO, in the relationship of AO to the circle and the torus, respectively. The motion of (Q) is always continuous, and, the effect of (P) on (Q)'s motion is continuous, and the effect of (A)'s motion on (Q) is continuous. But the transitions of the manifold from when (Q)'s motion is being determined by (Q) alone, to being determined by the effect of (P), to being determined by the connected effect of (A) and (P), are discontinuous. Yet as Archytas's construction implies, there exists a higher, cognitive continuity, that unifies, as if from outside, the discontinuities among these three manifolds of action. A similar point can be made from the standpoint of Gauss's geometrical proof of the fundamental theorem of algebra. From the perspective of formal algebra, the terms of an equation such as x^2+x+1=0 are distinguished from one another simply by how many times "x" is multiplied by itself. (2 for the first term, 1 for the second and 0 for the third.) But from the standpoint of physical geometry, the highest term indicates a degree of extension that determines the governing characteristics of the entire function. In other words, in the above example, we are not adding a square to a line to a point. Rather, the "x^2" is an area (x by x) and so is the "x" (x by 1)and so is the "1" (1by 1). But if those same terms are subsumed into a cubic equation, x^3+x^2+x+1=0, they are all transformed into volumes. (For example, the "x^2" in the cubic equation is no longer an x by x area, but is transformed into a x by x by 1 volume.) In his proof of the fundamental theorem of algebra, Gauss created a higher geometry that expressed the continuity within any single algebraic power with respect to that power's discontinuous relationship to all other algebraic powers. Thus, Gauss's higher geometry expresses the discontinuities between the algebraic powers, {cognitively} as an effect of a simultaneous continuity. What Gauss accomplished with his studies of algebraic powers, Riemann achieved for that succession of transcendental species of powers, known as "Abelian functions".

The Circle in the Complex Domain

Before turning directly to Riemann's accomplishment, let us secure our footing by looking at Gauss's earliest discovery the division of the circle, from the vantage point of Riemann's work. As was already known to the Pythagorean geometers, the principle on which the division of the circle into equal parts depends, can not be found in the circle itself. As Kepler summarizes this knowledge in the first book of the Harmony of the World, the division of the circle into equal parts by magnitudes that are constructable (knowable) from the circle and its diameter is limited--a characteristic that from the Pythagoreans onward was recognized as the futility of achieving any universal knowledge from the so-called straight-edge and compass constructions. Though this limitation was recognized from the Pythagoreans through Kepler, it was not until Gauss looked at the matter from the standpoint of the complex domain, that the{ principle} on which this limitation is based was known. Since we have treated Gauss's discovery of the division of the circle in previous installments of this series and in numerous oral presentations, we focus here only on the general considerations. First we must extricate our mind from its attachment to the idea that a circle is a self- evident object in visible space, which we divide into "n" visible pieces to form an "n" sided polygon. Instead, think of a circle divided into "n" distinct parts as the result of a single generating principle in which the "n" parts are intrinsic to manifold. This can be done when the circle is considered to be a special type of pathway in a manifold of action in which the pathways of least action are always logarithmic spirals. Such a manifold can be expressed by the complex exponential (See Figure 4.)

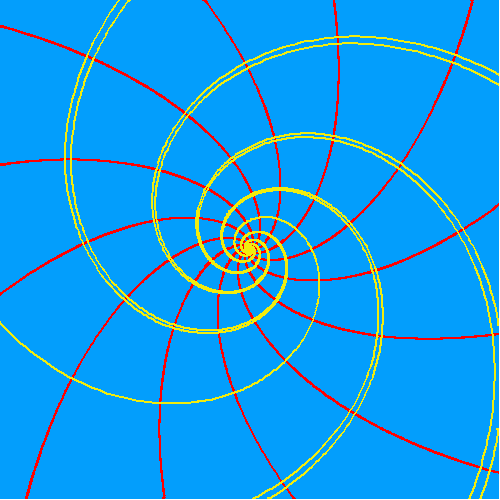
While there is an unlimited possibility for variation among these logarithmic spirals, depending on the rate of growth of the spiral, such unlimited possibility is {not} unbounded. The minimum-maximum relationship bounding this manifold is expressed by the spirals with maximum rotation and minimum extension, which are called "circles" and the spirals of maximum extension and minimum rotation, which are called, "straight lines". The metric relation intrinsic to this "exponential-logarithmic" manifold can be expressed by the relationship between how a change in position along a spiral pathway is measured by an angle and how that same change in position is measured by a change in the length of the corresponding arc or radial line. (Note that the arc and radial line are both spiral pathways.) (See figure 5).

This relationship is that equal angles correspond to exponential changes in arc length. Thus, an increase of the angle by "n" increases the corresponding arc length by the "nth" power. (For example, a change in position measured by a doubling of an angle corresponds to squaring of the arc length along the spiral path.) (See Figure 6)

However, for that special type of logarithmic spiral known as a circle, the equal angles correspond to equal arcs. But the intrinsic logarithmic characteristic of the spiral is, nevertheless, embedded in the circle as well and it is expressed in the relationship of the angle to the sine and the cosine, when that relationship is expressed in the complex domain. (See Figure 7).

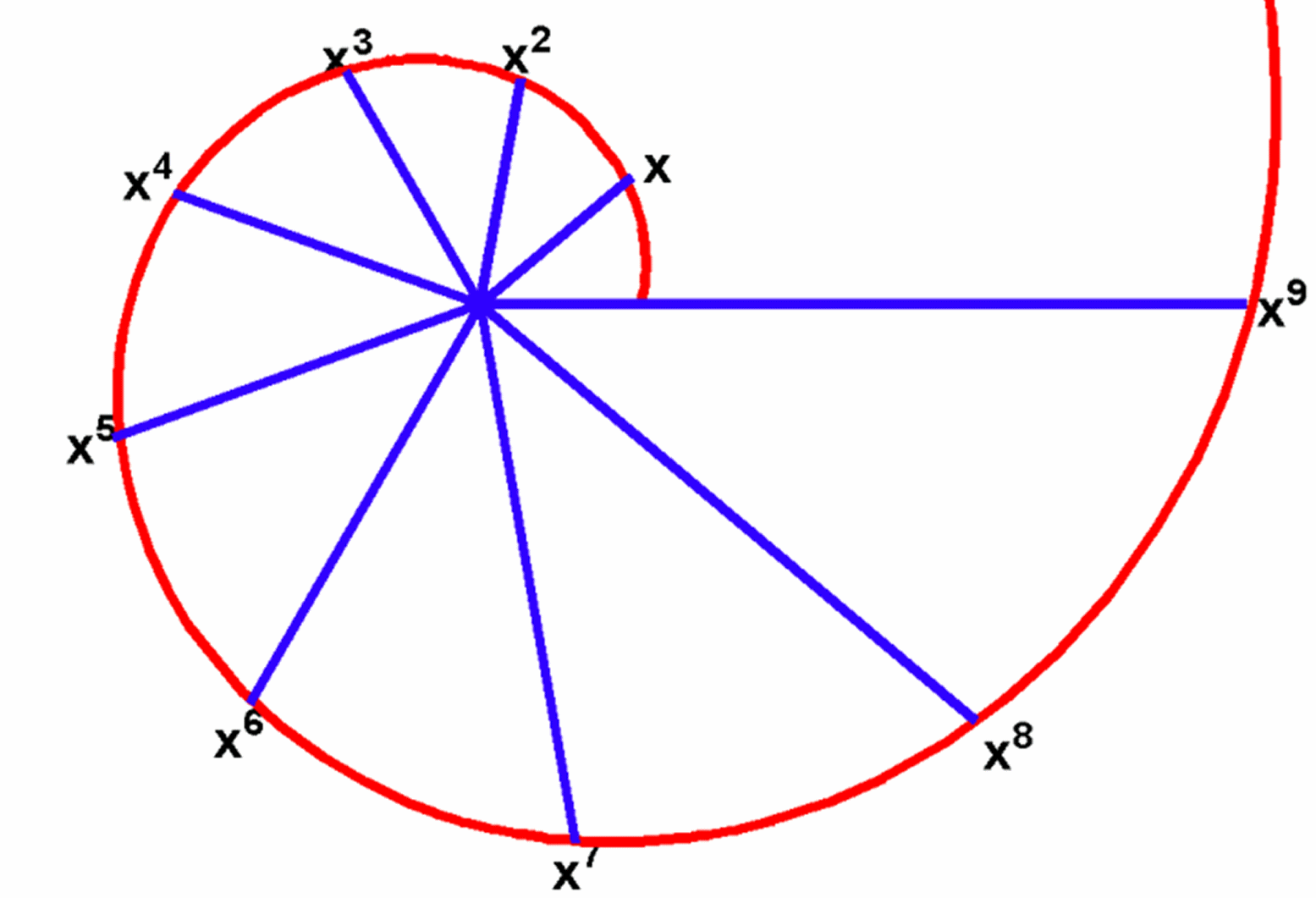
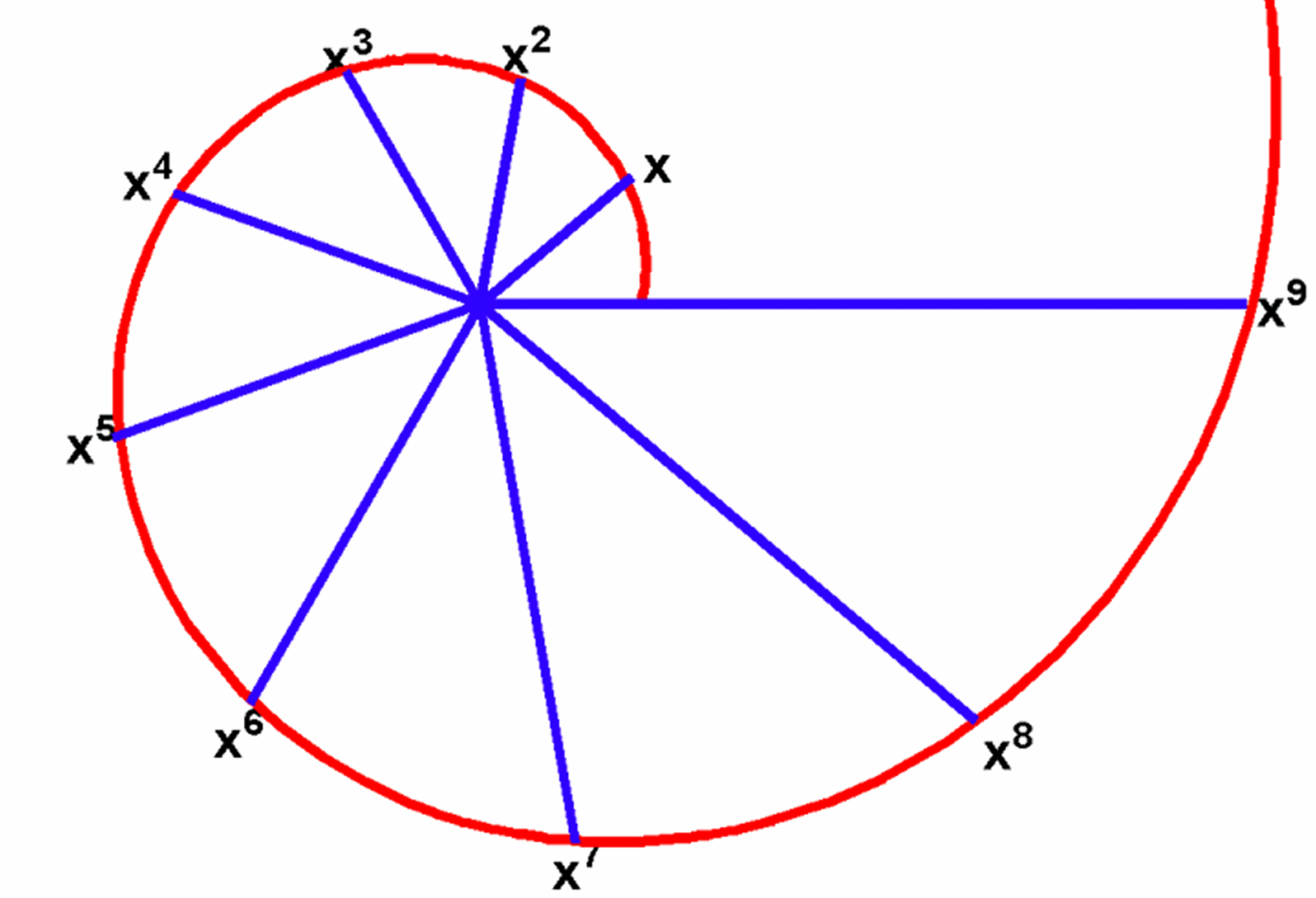
Thus, dividing a circle into "n" parts corresponds to finding "n" powers within an angle of 360 degrees. This principle can be expressed by the simple algebraic expression, "x^n-1=0". This can be grasped intuitively by looking at animated figure 8 which depicts the complex cubing function. In the animation, the cubing function triples the angles swept out in the left panel, the effect of which is depicted in the right panel.

Thus, the vertices of a regular triangle are the points of discontinuity where the action on the left sweeps out one complete circle on the right. This is depicted in the animation by a change of color. For what follows it is useful to also look at this same action by inversion as depicted in animated figure 9.

Here we see the black circle on the right, simultaneously, sweeping out three unique circular segments on the left. Seen in this way, the vertices of an "n" sided polygon can be understood as "n" discontinuities produced by the continuous action of an algebraic function of the "nth" power. This gives rise to a new form of the same paradox cited above with respect to Plato's investigation of the incommensurability of the line, square and cube. In the complex domain, an "nth" power algebraic function produces, by a continuous process,"n" discontinuities, but it can never produce "n+1" such discontinuities. The transition from "n" to "n+1" is itself discontinuous, but it signifies a higher type of discontinuity, than that expressed by the vertices of a regular polygon. Further, though each lower power signifies a manifold containing "n" discontinuities, the succession of higher discontinuities in the transitions from "n" to "n+1" to "n+2", etc. , when considered simultaneously, imply a cognitively generated new, higher, type of continuity. Now, look at this paradox further from the standpoint of Cusa's determination of the transcendental nature of the circle. If an "n" sided polygon is produced by an algebraic function of the "nth" power, the power of the function that produces the circle must be infinite, and therefore, outside the domain of algebra. It exists in the domain that Leibniz called transcendental, which subsumes all possible algebraic relationships. Thus, the transcendental power that generates the circle, generates, simultaneously, the discontinuous manifold of all possible regular polygons.

A Higher Discontinuity

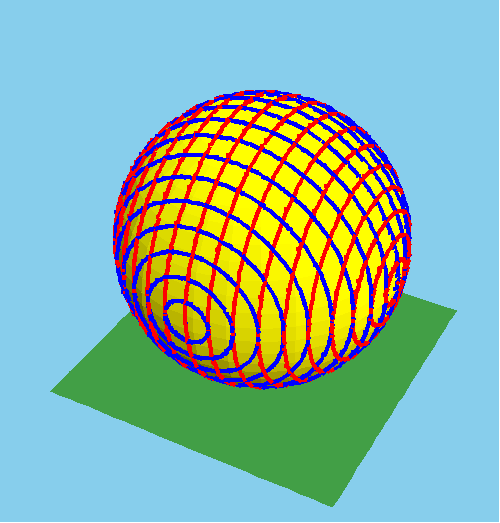
While the discontinuous manifold of the algebraic powers is bounded by the simple transcendental power that generates the circle, our investigations of the physical universe demand that we find conceptions of manifolds of still greater power. The path on which to look for such conceptions was discovered by Gauss, Abel, Dirichlet and Jacobi, but its illumination we owe to Riemann. That path is not free of obstacles. In fact, it is the obstacles we seek and with Riemann's beacon we can find them. The first obstacle we seek is that which bounds the simple transcendental power that generates the circle. But to find it in the visible circle itself would be futile, as the visible circle appears to be obviously unbounded. Nevertheless, that boundary, of the domain of simple transcendentals, emerges clearly when we look, with the aid of Riemann's surfaces, at the circle as an inversion from the circular functions. As was illustrated in the last installment of this series, the essential characteristic of the simple transcendental functions is that they are simply-periodic. This characteristic is evident from the visible expression of the circular functions, for as the sine goes from 0 to 1 to 0 to -1, the angle rotates counterclockwise around a circle. Thus, while the circular angle has an unbounded potential, the circular sine is always bounded between 1 and -1. An insight into the importance of this can be gleaned from posing this relationship as an inversion. The question, "what is the sine of an angle?" has only one answer, but the question, "what is the arc that has this sine?" has an unlimited number of answers. The crucial implications of this simply-periodic characteristic can be most easily seen by, as we did above, looking first at the complex circular functions from the more general expression, the complex exponential, which, as we saw, is a manifold of action in which the least-action pathways are logarithmic spirals. It is evident from the intrinsic geometry of the spiral, especially when viewed from the standpoint of the Riemann sphere, that this manifold has two singularities, which on the Riemann sphere are the north and south pole. (See figure 10.)

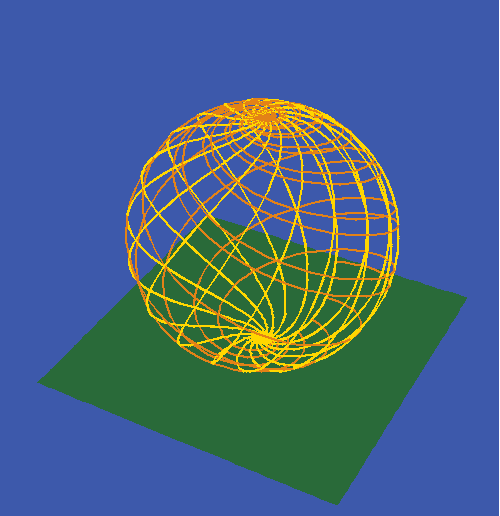
In this manifold, circles, are simply those spirals that rotate around the poles but do not extend to them. "Straight" lines are simply the spirals that extend to the poles but do not rotate. Like all the other spirals in this manifold, these limiting cases are also bounded by the poles. Riemann recognized that a manifold with two singularities was intrinsic to the characteristics of spherical geometry. Consequently, he defined spherical geometry as the geometry of manifolds of two singularities. However, as was also illustrated in the last installment, the singularities exemplified by the poles of the complex exponential in a Riemann's surface, are a special type of singularity, which Riemann called, "branch points." This is due to the fact that since the complex exponential is periodic in one direction (the "latitudinal" direction), each rotation must add another layer, or, "branch" to the manifold. (See figure 11).

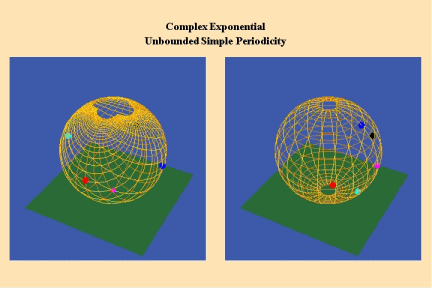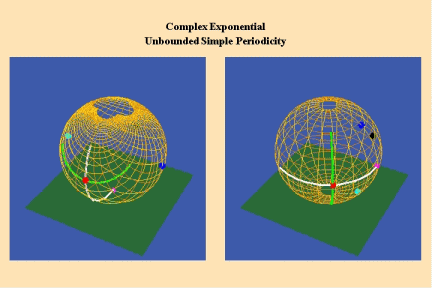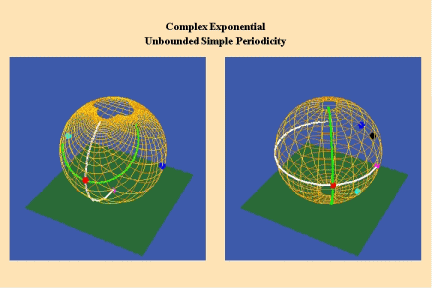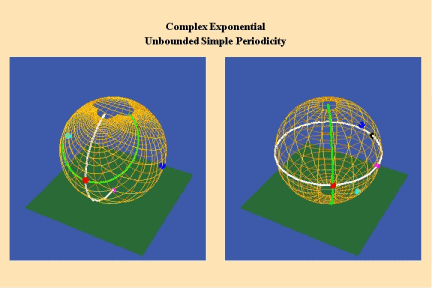
Thus, the Riemann surface that depicts the manifold of the complex exponential is a sphere with an unlimited number of layers, joined at the north and south pole. A "branch cut" is made from one pole to the other. Any action that crosses this cut, crosses onto the next layer. Consequently, action in the "latitudinal" direction, necessarily crosses the branch cut, while action in the "longitudinal" direction necessarily does not. From this perspective we can now understand the circular functions as a complex exponential whose branch points have been rotated from the poles, to the points representing 1 and -1 on the equator. (The hyperbolic functions are simply the simple transcendentals with branch points at i and -i, thus completing the simple transcendental domain.) The branch cut now extends from these points on the equator to the north pole. (See Figure 12).

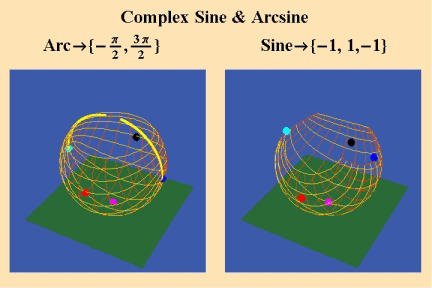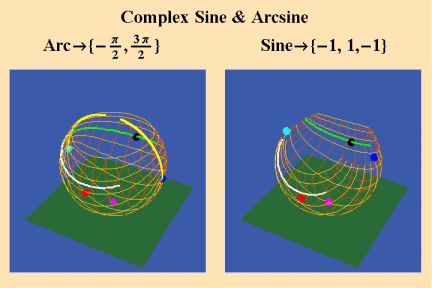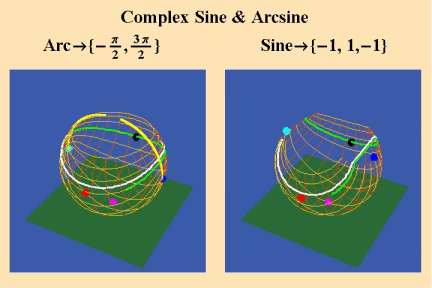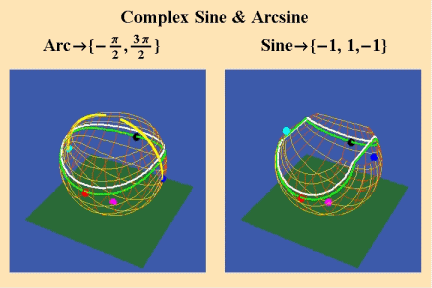
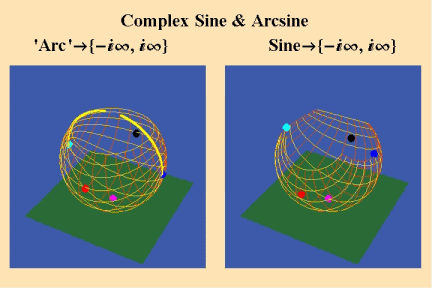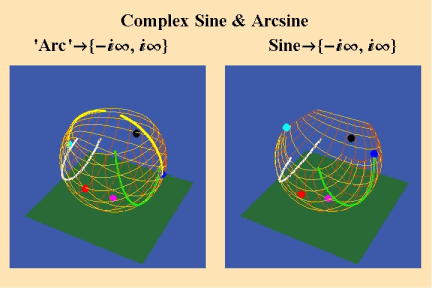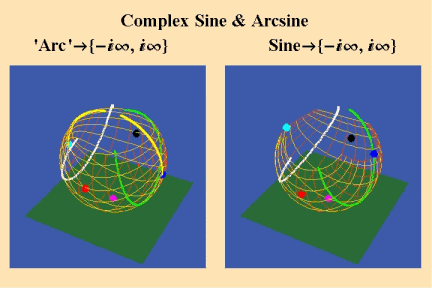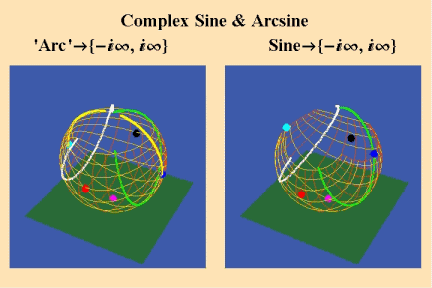
Just like the complex exponential, any action that crosses this branch cut, crosses over onto another layer of the Riemann's surface. A further insight into this characteristic is gained by looking more closely into the Riemann's surfaces for the circular functions. In animated figure 13 the left panel shows a rotation going from -90 degrees to 90 degrees while the right panel shows the sine going from -1 to 1.

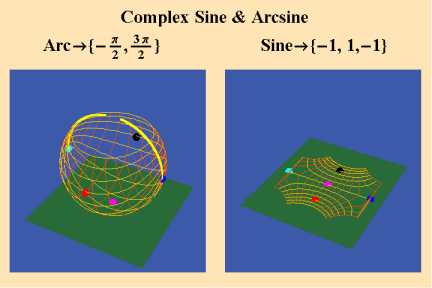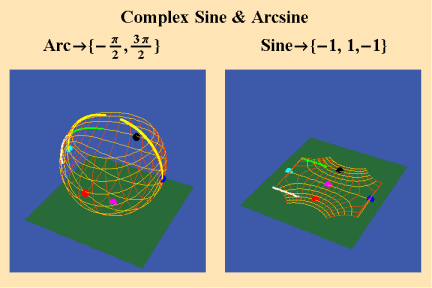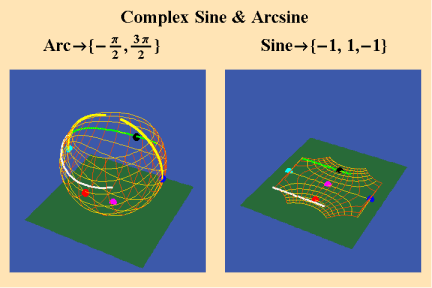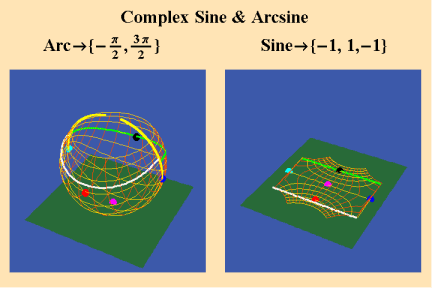
In animated figure 14 this rotation is continued, but as it starts back toward the beginning it crosses the branch cut. This produces a corresponding discontinuous jump in the sine as it moves back from 1 to -1.

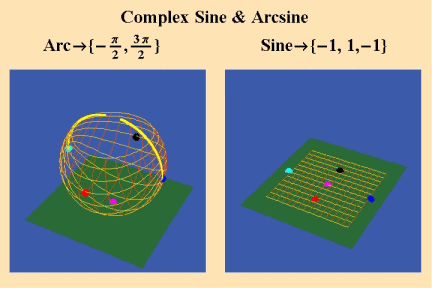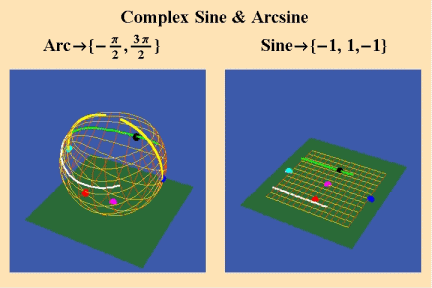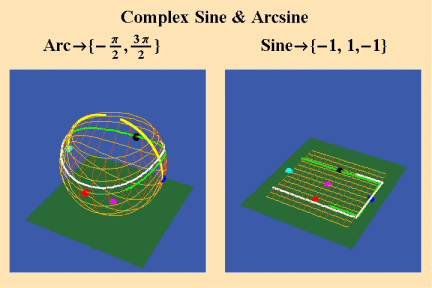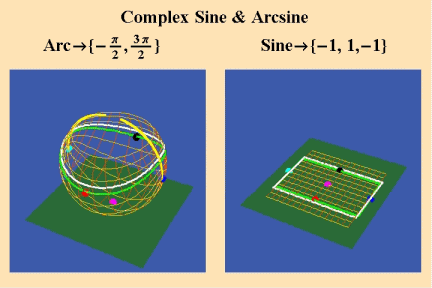
However action in an orthogonal direction does not cross the branch cut and is consequently, unbounded. (See animated figure 15).

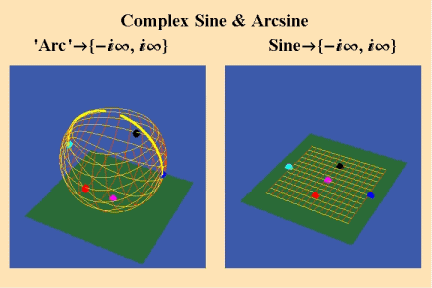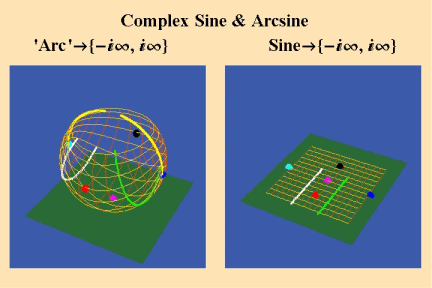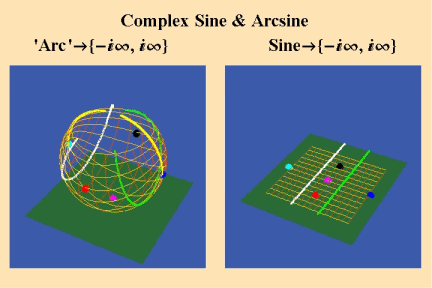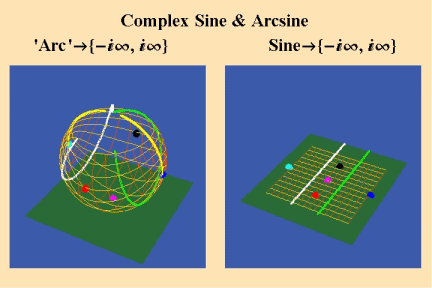
This difference can be seen more completely when viewed from the standpoint of the Riemann sphere. (See animated figures 16).

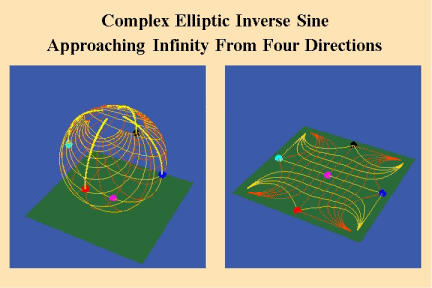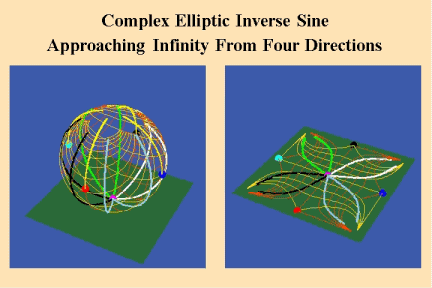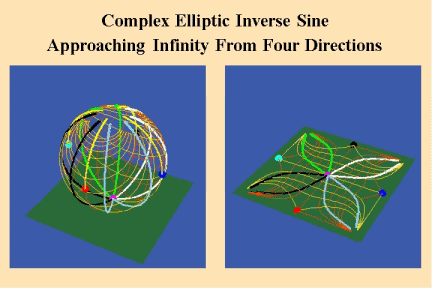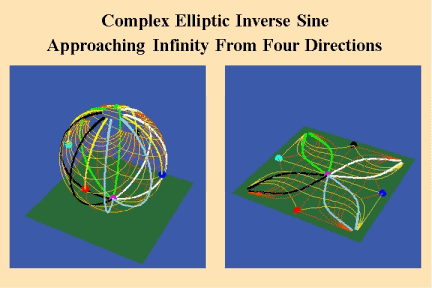
Armed with this Riemannian view of simple transcendentals we can see that the essential characteristics of simply periodic manifolds are expressed by the intrinsic existence of two branch points. Such manifolds arise from the investigation of physical processes which can be understood as the effect of the transcendental action of only one principle. For example, in simple uniform circular motion, the sine is transcendental with the arc, but the arc is uniform with the angle. Or, in the case of the catenary, the gravitational effect varies but the tension is always constant. However, as the "Kepler Problem" illustrates, in non-uniform physical motion, two or more transcendental principles are acting, connectedly and simultaneously, to produce the perceived physical effect. For example, in the case of elliptical action, the sine is incommensurable with the arc, but the arc is also incommensurable, according to a different principle, with the angle. (A similar type of double incommensurability arises when expressing the relationship of the varying period of a circular pendulum the angle formed by the string and the vertical.) Gauss, Abel and Jacobi all recognized that these types of physical action could only be expressed by a higher type of transcendental a transcendental function that had the power to unify two connected but distinct transcendental actions. However, a clear and elementary understanding of these transcendentals is only possible from the vantage point of Riemann's surfaces. To intuitively grasp the concept of these higher transcendentals, let us investigate how the elliptical transcendentals are distinguished from simple transcendentals by the characteristics of their corresponding Riemann's surface. As Gauss demonstrated for the case of the lemniscate, the characteristic of the elliptical transcendentals are distinguished by their double periodicity. Riemann showed that this characteristic of double periodicity implied a distinctly different manifold of action than the spherical manifold of the simple transcendentals. This distinction comes to light, not in the visible shape of the circle and ellipse, but only through investigating the different characteristics of the Riemann's surfaces that correspond to the transcendental functions that generate them. As we will illustrate by animation, a manifold that is capable of permitting doubly periodic action must be toroidal, as distinguished from the spheroidal manifold capable of permitting simply periodic action. The first insight can be gained by looking at doubly periodic action from the standpoint of Riemann's sphere. In animated figure 11 we saw a Riemann's sphere depicting a simply periodic manifold. In the left panel both the white and green paths approach the infinite from two orthogonal directions while in the right panel, the white path becomes periodic while the green remains unperiodic. In animated figure 17, depicting elliptical transcendentals, both the white and the green paths become periodic in the right panel.

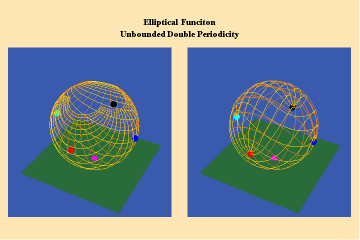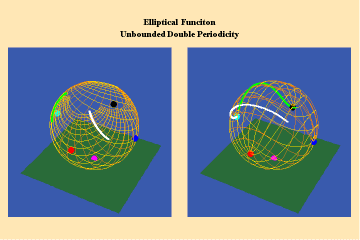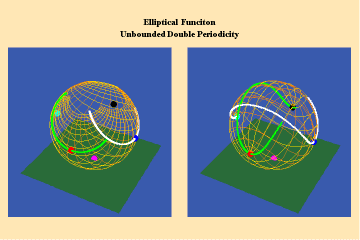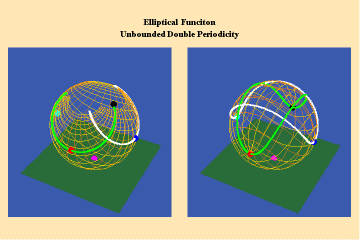
Riemann showed that such doubly periodic action cannot occur in a manifold with the same spherical characteristics as the manifold of the simple transcendentals. In a simply periodic function, as we saw in the case of the complex sine and complex exponential, there is only one set of closed, periodic curves and these converge on two points (the poles in the case of the complex exponential and 1 and -1 in the case of the complex sine.) (See Figure 18).

But, in a doubly periodic function there are two orthogonal sets of closed curves, and each set converges on a different pair of points. (In the case of the lemniscate those points are (1, -1) and (i ,-i) (See Figure 19).

Thus, a manifold that is capable of permitting doubly periodic action must have four branch points and two branch cuts connecting each pair of branch points. (See Figure 20).

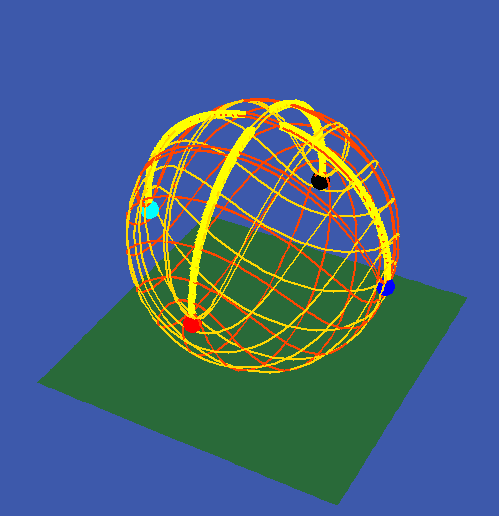
From this it can be seen, as Riemann insisted, that a manifold with four branch points {must} be of a fundamentally different nature than a manifold of only two branch points. To begin to get a sense of why that is the case, look at the animated figure 21.

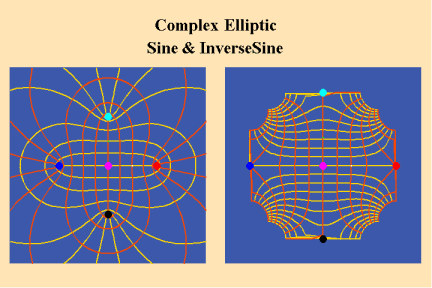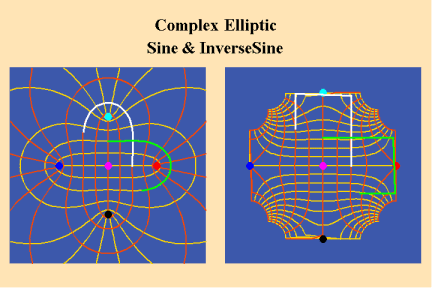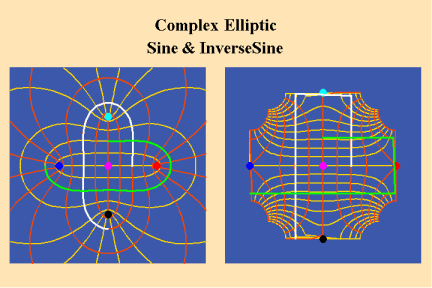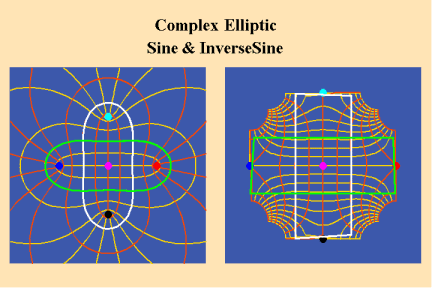
In the left panel we see the two distinct rotations of the elliptical function and the on the right we see their corresponding periods. In the animation, one period is bounded horizontally while the other is bounded vertically. Thus, the manifold of a doubly-periodic elliptical transcendental must be doubly self-bounded. A sphere, however, is not doubly self-bounded. But, Archytas's torus is. (See Figure 22.)

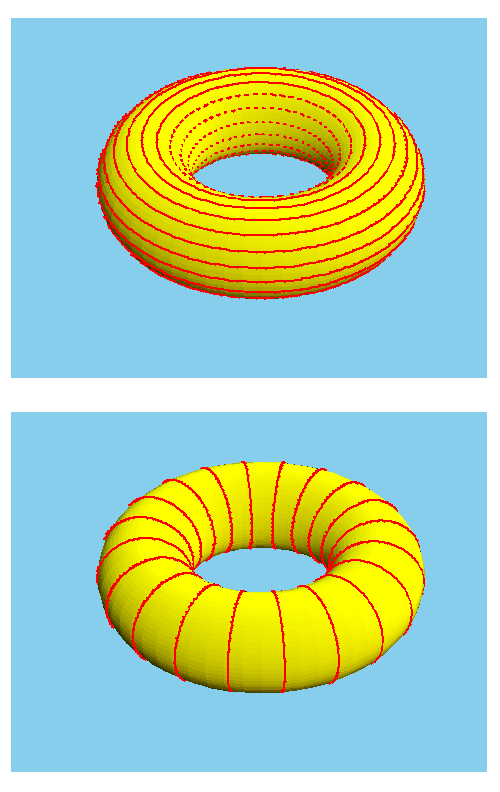
The further characteristics of such a doubly bounded manifold can be intuitively grasped by animated figure 23.

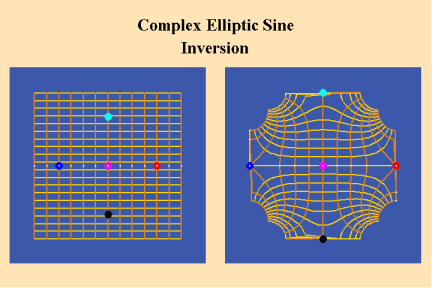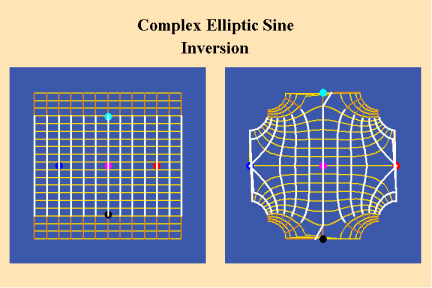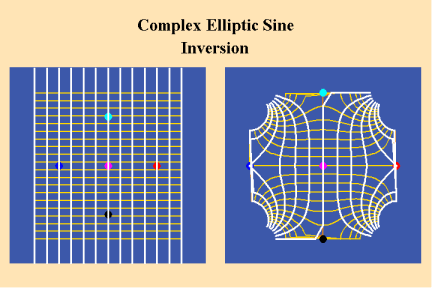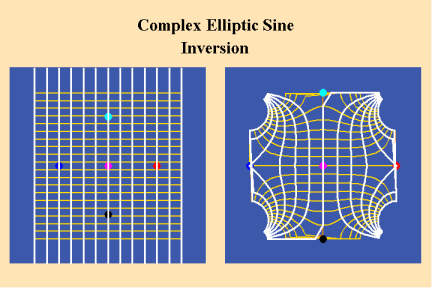
In the left panel we see lines moving in the vertical direction and in the right panel we see that their images must veer towards the horizontal as they approach the horizontal boundaries. Similarly, in animated figure 24, the lines moving in the horizontal direction in the left panel, veer towards the vertical, in the right panel, as they approach the vertical boundaries.

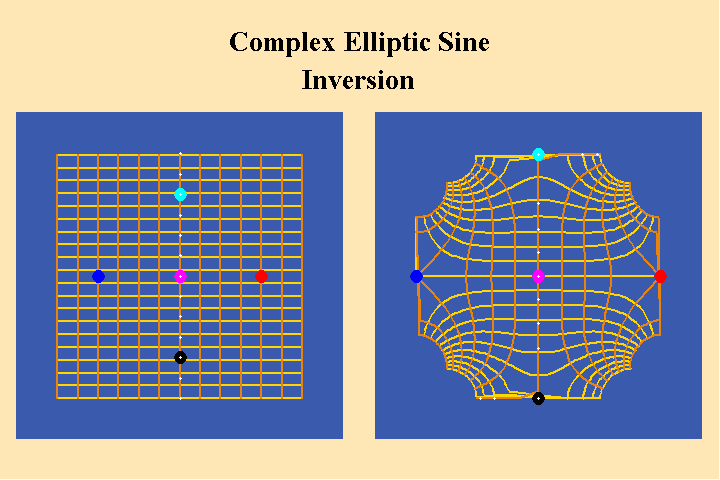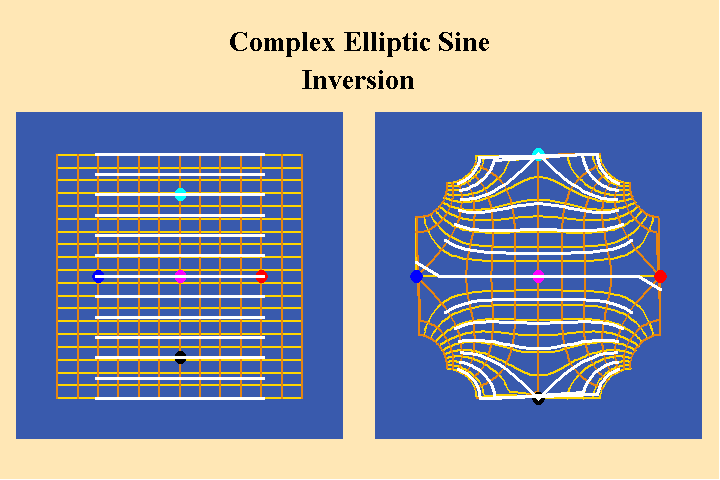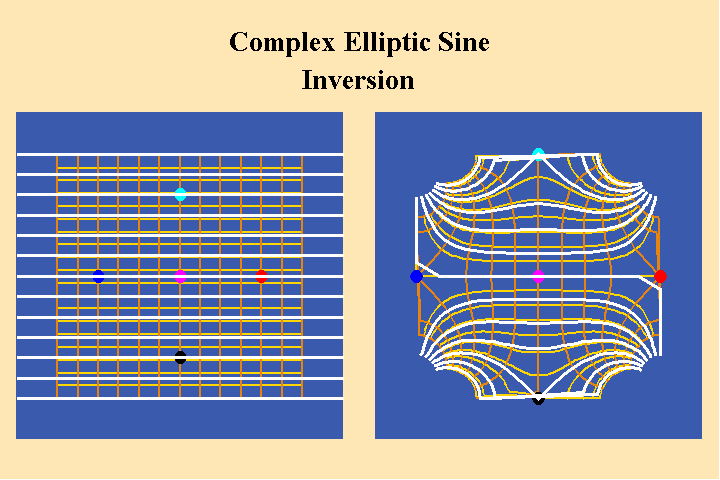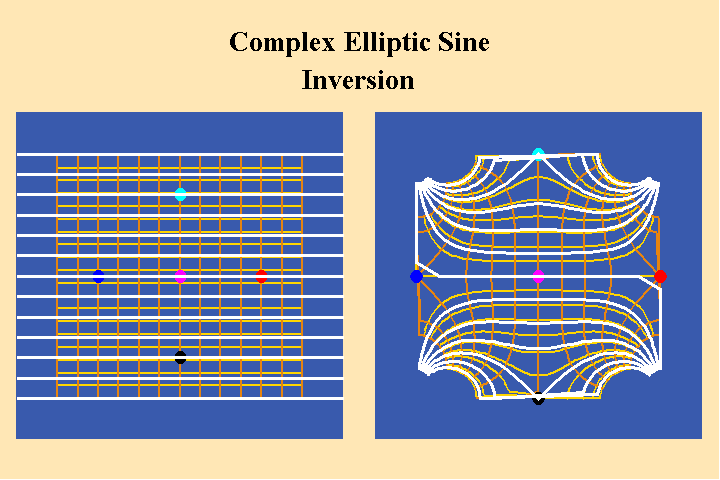
This effect produces four points of convergence, one at each corner of the square. These points of convergence are the "poles" of the manifold. Thus, a doubly-periodic elliptical manifold must be a manifold with four poles. Animated figure 25 shows the futility of attempting to represent such a manifold by spherical geometry.

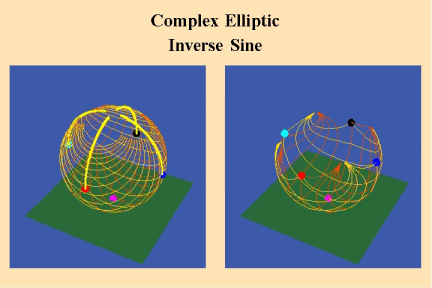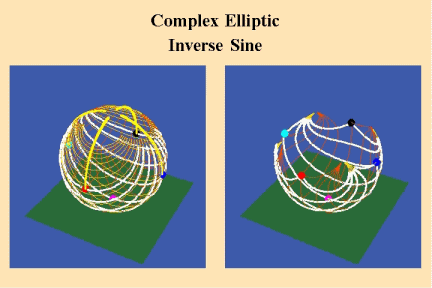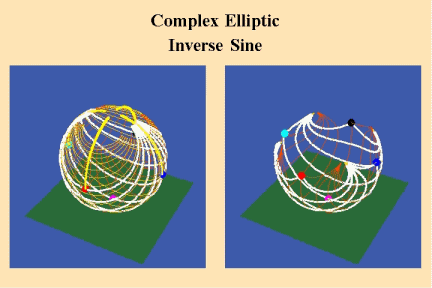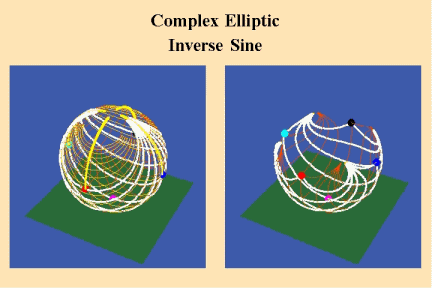
As Riemann emphasized, a manifold with four such poles is fundamentally different topologically than a sphere. The investigation of this characteristic, Riemann identified, belongs to the domain of Leibniz's "Analysis Situs", which seeks to discover the fundamental characteristics that are intrinsic to a manifold. Under this way of thinking, we can see, as Riemann did, that the capacity to sustain a certain density of singularities is just such an intrinsic characteristic. This capacity is determined by the number of principles acting in the manifold. Applying Dirichlet's Principle, Riemann demonstrated that this characteristic the power of the manifold to sustain singularities-- determines all possibility for action within the manifold. An increase in power must correspond to a change in these topological characteristics. Such a change cannot come from within the manifold itself, but only by the addition of a new principle, from outside, {implying the necessary existence of a higher manifold}. This change, from the spherical to the toroidal requires a change in our depiction of the elliptical functions. In the following animations the spherical action is depicted on the left and the toroidal action as projected onto a plane on the right. Think of the square in the right panel as an "unfolded" torus. In animated figure 26 we see how four paths that approach the single pole of the sphere are mapped onto the four distinct poles of the unfolded torus.

In animated figure 27 we see how a circular path through the four branch points maps to a square on the unfolded torus.

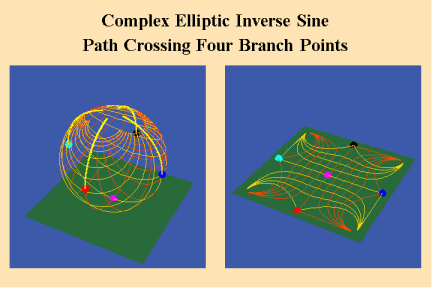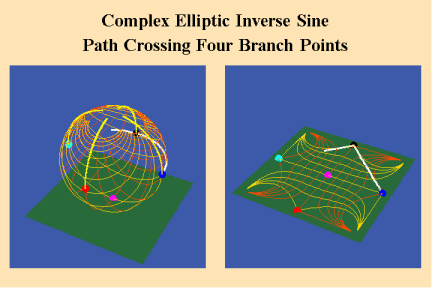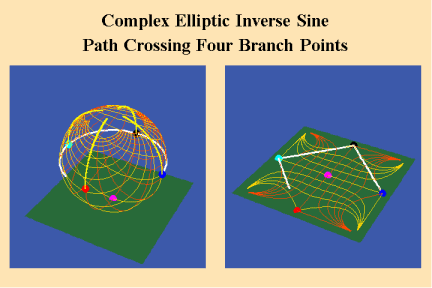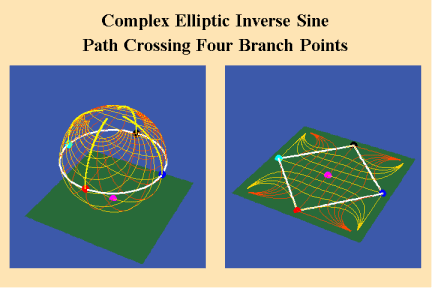
In animated figure 28 we see how a circular path crossing all four branch cuts maps onto the unfolded torus, as a curve with four distinct discontinuities.

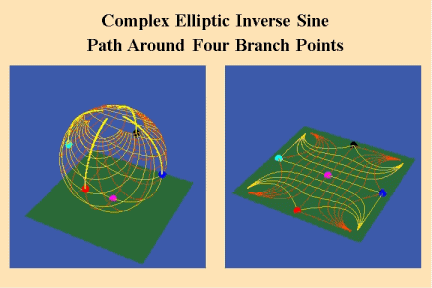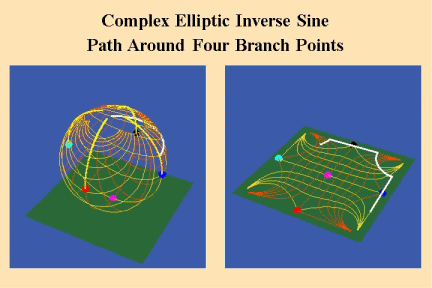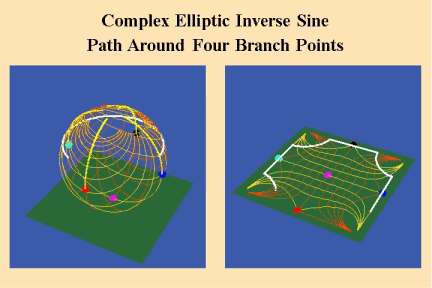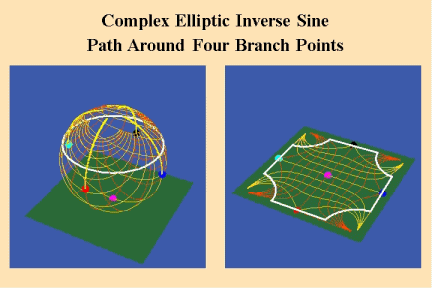
Each time the path crosses a branch cut on the sphere, its image crosses a discontinuity on the torus. This sets the stage for the discontinuous jump into the next highest transcendental function the hyper-elliptical. We will leave it to the next installment to more fully develop its characteristic, but from animated figure 29 we can see that it has eight branch points, eight branch cuts and eight poles.

Gauss and Riemann both understood that a self-bounded manifold with this capacity must be negatively curved and, in principle, cannot be represented directly within the positive curvature of visible space. Nevertheless, its shadow can be projected into the visible domain as indicated by the negatively curved octagon in the animation. Thus, the domain of Abelian transcendentals, as seen from Riemann's vantage point, is a discontinuous manifold of continuous manifolds, each of which is characterized by the capacity to sustain a greater number of discontinuities. This {Discontinuum}, nevertheless, has a continuity, intrinsic not to itself, but to the cognitive act from which it has been conceived.