"Don't tell me how the universe is constructed," protested an early 17th-Century ancestor of today's Baby Boomer, "I just need to know when Mars will be in Leo, when Saturn will be in opposition to the Sun, and when Jupiter will align with the Moon. Armies are massing, and on good information, I've been told, that when the stars are such, the war begins. When armies amass, I make cash. Dice, whiskey and whores. I get a cut of them all. But once the fighting starts, it's too late to speculate. I need to know now, what's in store. Buy cheap and sell dear, they say. It's best if I tell the others to sell. But, it only works, if they think the information that I've got, they have not."

"My dear son," the old man said, after a long pause, "You have to be human to understand matter."

Perhaps Kepler never encountered an interlocutor as crude as this. Nevertheless, he must have confronted something similar:

"Yet, alas, of what great goods do miserable mortals despoil one another, by their shameful itching for quarrels. How profound an ignorance of their fate overwhelms them, as they have deserved. With what deplorable perverseness do we rush into the midst of the flames, in fleeing from the fire," he wrote in 1621, three years after the eruption of the Thirty Years War.

"Would that even now indeed, there may still, after the reversal of Austrian affairs which followed, be a place for Plato's oracular saying. For when Greece was on fire on all sides with a long civil war, and was troubled with all the evils which usually accompany civil war, he was consulted about a Delian Riddle, and was seeking a pretext for suggesting salutary advice to the peoples. At length he replied that, according to Apollo's opinion Greece would be peaceful if the Greeks turned to geometry and other philosophical studies, as these studies would lead their spirits from ambition and other forms of greed, out of which wars and other evils arise, to the love of peace and to moderation in all things."

Such was Kepler's introduction to the second edition of his Mysterium Cosmographicum, published 25 years after its first appearance in 1595. The intervening period was marked by an escalation of the Venetian-led religious wars, that had by then been ongoing since 1513, and was accompanied by an orchestrated, popular rise in superstition and witchcraft, in which Kepler even saw his own mother victimized in a witch trial. Meanwhile, Kepler had extended the discoveries contained in his first work, elaborating the principles underlying the motions of the solar system, and, composing those principles into one unified function, of the type, characterized by Gauss nearly 200 years later, as "hypergeometric".

"Astronomy has two ends," Kepler wrote in the "Epitome of Copernican Astronomy", "To save the appearances and to contemplate the true edifice of the world." For Kepler the principles necessary for the latter would satisfy the requirements of the former. Numerous efforts to save the appearances had been attempted previously, and all had turned up wanting. All suffered from a common flaw, not in the sophistication of their geometrical constructions, but in their underlying intent, which was simply to predict, and not to know.

To know, instead of simply to predict, requires, "the discipline which discloses the causes of things, shakes off the deceptions of eyesight, and carries the mind higher and farther, outside of the boundaries of eyesight. Hence, it should not be surprising to anyone that eyesight should learn from reason, that the pupil should learn something new from his master which he did not know before...," Kepler wrote in the "Epitome".

The "hypergeometric" type of function that Kepler composed, is, thus, comprised of a set of physical constraints, that, taken as a One, permit only those motions that physically occur, to occur. Each constraint is itself a function, a sort of special case, of the more generalized "hypergeometric" function that characterizes the ordering of the Solar System.

Exemplary is Kepler's discussion of the constraint concerning the number of the planets, as stated in the "Epitome":

"This worry has been resolved, with the help of God, not badly. Geometrical reasons are co-eternal with God -- and in them there is first the difference between the curved and the straight line. Above (in Book 1) it was said that the curved somehow bears a likeness to God; the straight line represents creatures. And first in the adornment of the world, the farthest region of the fixed stars has been made spherical, in that geometrical likeness of God, because as a corporeal God -- worshipped by the gentiles under the name of Jupiter -- it had to contain all the remaining things in itself. Accordingly, rectilinear magnitudes pertained to the inmost contents of the farthest sphere; and the first and the most beautiful magnitudes to the primary contents. But among rectilinear magnitudes the first, the most perfect, the most beautiful, and most simple are those which are called the five regular solids. More than 2,000 years ago Pythagoreans said that these five were the figures of the world, as they believed that the four elements and the heavens--the fifth essence--were conformed to the archetype for these five figures.

The Five Regular Solids

"But the true reason for these figures including one another mutually is in order that  these five figures may conform to the intervals of the spheres. Therefore, if there are five spherical intervals, it is necessary that there be six spheres; just as with four linear intervals, there must necessarily be five digits. "

Kepler's Intervals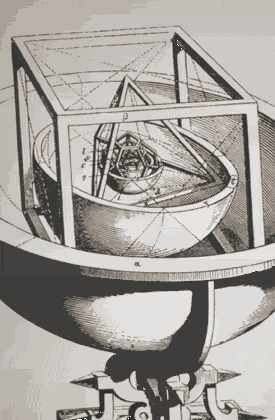

Thus, the constraint that determines the number of planets and the general size of their orbits, is a special case of the "hypergeometric" function, and reflects the incommensurability of the straight to the curved. As we have shown in other pedagogical discussions, there is a different characteristic between action on a flat (straight) surface and a spherical one. The five Platonic solids represent the totality of all possible equal divisions of a sphere, while, a flat surface permits an entirely different set of tilings. Further, the characteristic periodicity of a flat surface is four-fold, while (as Kepler's contemporary Napier showed in his studies on the pentagramma mirificum) the characteristic periodicity of a spherical surface is five. The correlation between this geometrical paradox, and the physical nature of the solar system, is evidence that the "hypergeometric" function ordering the solar system is curved, i.e., anti-Euclidean.

Yet, spherical action is not sufficient by itself to characterize planetary motion. While the total periodic time of each planetary orbit is constant, "It, nevertheless, is of irregular speed in its parts; and it makes the planet in one fixed part of its circuit digress rather far from the Sun, and in the opposite part come very near to the Sun; and so the farther it digresses, the slower it is; and the nearer it approaches, the faster it is.... For astronomy bears witness that, if with our mind we remove all deceptions of sight from that confused appearance of the planetary motion, the planet is left with such a circuit that in its different parts, which are really equal, the speed of the planet is irregular ... astronomy, if handled with the right subtlety, bears witness in this case that the routes or single circuits of the planets are not arranged exactly in a perfect circle but are ellipses."

These physical constraints, governing the irregular motion of the planets, are also a special case of that same generalized "hypergeometric" function, encountered peviously. As in the case of the physical constraints reflected in the Platonic solids, the difference between the straight and the curved are reflected here. While the motion of the planet within its orbit is constantly changing, the ratio of the area swept out between a line connecting the planet and the Sun, to the time elapsed, is always constant. Similarly, even though the sizes of the planetary orbits, and the periodic times are obviously different for each planet, the ratio between the square of the time and the cube of the mean distance from the Sun, is the same for all planets. These so-called second and third laws of Kepler, are thus, special cases of the "hypergeometric" function governing planetary motion. The one ratio is a constant within each orbit, the other across the orbits. (These matters were treated at some length in chapters five, six and seven of the Fidelio article on Gauss' determination of the Ceres orbit, and will form the jumping off point for future investigations).

Similarly, the harmonic relationships between the extreme angular velocities of adjacent planets, that correspond to musical intervals, is another special case, of this generalized "hypergeometric" function.

"But, Galileo made it all so simple," the Baby Boomer's ancestral soul-mate complains. "I like his way better."

Kepler certainly faced this problem: "As regards the academies, they are established in order to regulate the studies of the pupils and are concerned not to have the program of teaching change very often: in such places, because it is a question of the progress of the students, it frequently happens that the things which have to be chosen are not those which are are most true but those which are the most easy."